1.7 Selecting Procedures for Determining Limits
7 min read•january 9, 2023
ethan_bilderbeek
Anusha Tekumulla
ethan_bilderbeek
Anusha Tekumulla
AP Calculus AB/BC ♾️
279 resourcesSee Units
Selecting Procedures for Determining Limits
🎥Watch: AP Calculus AB/BC - Algebraic Limits
As we have previously discussed, there are several methods for determining limits: direct substitution, factoring, and trigonometric identities. But how do you know when to use each of these methods? The flowchart below provides a useful guide for selecting the appropriate method for determining a limit.
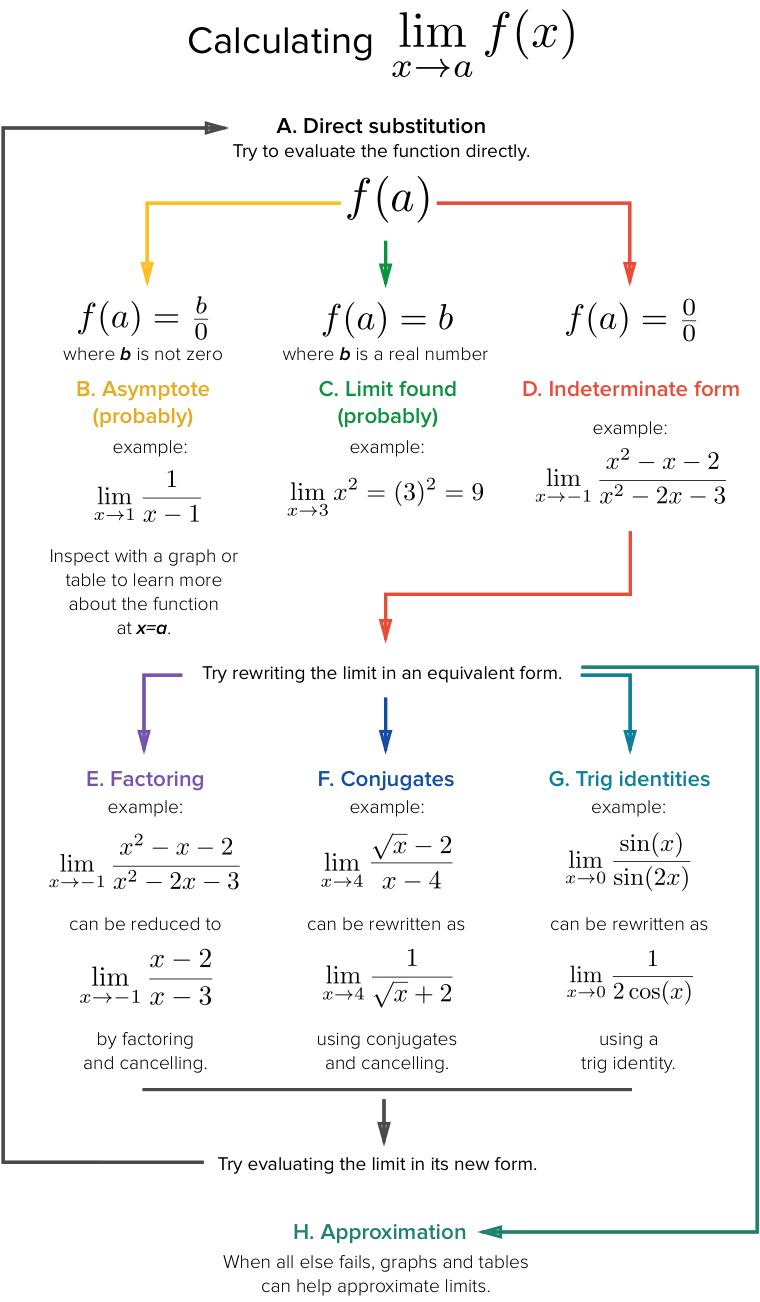
Khan Academy
The first step in determining a limit is to use direct substitution. This is the simplest and easiest method, and should always be tried first. When using direct substitution, there are three possible outcomes:
If you get a number as a result of direct substitution, this is most likely the limit. However, it is always a good idea to confirm this by looking at the graph of the function around the x value in question. This can help you ensure that the limit is accurate.
If you get an expression of the form b/0, where b is not equal to 0, then you most likely have an asymptote. An asymptote is a line that a graph approaches, but never touches. After confirming that there is an asymptote, you can conclude that the limit does not exist.
If you get an expression of the form 0/0, you have an indeterminate form. Indeterminate forms are expressions that cannot be simplified to a single number or infinity, and include expressions such as 0/0 and ∞/∞. In this case, you will need to use one of the other methods (factoring, conjugates, or trig identities) to determine the limit.
If you are unable to determine the limit using direct substitution, the next step is to try one of the other methods. Factoring involves simplifying the expression by factoring out common factors, while conjugates involve adding and subtracting conjugates to simplify the expression. Trigonometric identities involve using trigonometric identities to simplify the expression. These methods can help you simplify the expression and determine the limit.
If you are still unable to determine the limit using these methods, the next step is to approximate the limit. There are two methods for approximating a limit:
Graph the function on a calculator or computer, and look at the y-value as x approaches the limit. This can give you a good idea of what the limit is, but it is not an exact solution.
Use a table of values to plug in numbers that are very close to the limit, and see what the y-value approaches. For example, if you are trying to find the limit as x approaches 3, you can plug in 2.9, 2.99, 2.999, etc. and see what the y-value approaches. This method can give you a good approximation of the limit, but it is not exact.
Once you have an approximation of the limit, it is important to confirm its accuracy by checking the graph of the function. If the limit appears to be accurate, you can conclude that this is the limit of the function. If the limit does not seem accurate, you may need to try a different method or continue to approximate the limit more closely.
It is important to note that limits can be tricky, and there is not always a clear method for determining them. In some cases, you may need to use a combination of different methods or continue to approximate the limit to find the best solution. The key is to be patient, persevere, and keep trying different methods until you find a solution that works.
It is also important to understand that limits are a fundamental concept in calculus, and are used to define many important concepts such as derivatives and integrals. As such, it is essential that you become proficient at determining limits in order to succeed in your studies of calculus.
To practice determining limits, you can try working through examples from your textbook, online resources such as Khan Academy, or by using interactive tools such as calculators or computer programs. You can also try creating your own examples and working through them to get a better understanding of the process.
In summary, the key to selecting the appropriate method for determining a limit is to start with the simplest method (direct substitution) and then try more complex methods if necessary. If you are unable to determine the limit using these methods, you can try approximating the limit using a calculator or table of values. With practice and persistence, you will become proficient at determining limits and will be well on your way to success in your studies of calculus. Certainly! Here are some additional examples of selecting procedures for determining limits:
Example 1: Determine the limit as x approaches 3 of the function f(x) = (x^2 - 4x + 3) / (x - 3).
Using direct substitution, we plug in x = 3 to get:
f(3) = (3^2 - 4*3 + 3) / (3 - 3)
= (9 - 12 + 3) / 0
Since we get an expression of the form b/0, where b is not equal to 0, we know that we have an asymptote. We can conclude that the limit does not exist.
Example 2: Determine the limit as x approaches 1 of the function f(x) = (x^2 - x - 2) / (x - 1).
Using direct substitution, we plug in x = 1 to get:
f(1) = (1^2 - 1 - 2) / (1 - 1)
= (1 - 1 - 2) / 0
Since we get an expression of the form b/0, where b is not equal to 0, we know that we have an asymptote. We can conclude that the limit does not exist.
Example 3: Determine the limit as x approaches 4 of the function f(x) = (x^2 + 2x - 8) / (x - 4).
Using direct substitution, we plug in x = 4 to get:
f(4) = (4^2 + 2*4 - 8) / (4 - 4)
= (16 + 8 - 8) / 0
Since we get an expression of the form b/0, where b is not equal to 0, we know that we have an asymptote. We can conclude that the limit does not exist.
Example 4: Determine the limit as x approaches 0 of the function f(x) = sin(x) / x.
Using direct substitution, we plug in x = 0 to get:
f(0) = sin(0) / 0
Since we get an expression of the form 0/0, we know that we have an indeterminate form. We can use trigonometric identities to simplify the expression and determine the limit.
Using the identity sin(x) = 2sin(x/2)cos(x/2), we can rewrite the expression as:
f(0) = sin(0) / 0
= (2sin(0/2)cos(0/2)) / 0
= 2*(1*0) / 0
= 0/0
We are still left with an indeterminate form, so we can try using another identity. Using the identity cos(x) = 1 - 2sin^2(x/2), we can rewrite the expression as:
f(0) = sin(0) / 0
= (2sin(0/2)cos(0/2)) / 0
= 2*(10) / 0
= 0/0
= (2sin(0/2)(1 - 2sin^2(0/2))) / 0
= 2(1*(1 - 2*(0^2))) / 0
= 2*(1*(1 - 0)) / 0
= 2*(1) / 0
= 2/0
Since we get an expression of the form b/0, where b is not equal to 0, we know that we have an asymptote. We can conclude that the limit does not exist.
Example 5: Determine the limit as x approaches 0 of the function f(x) = x^2 / x.
Using direct substitution, we plug in x = 0 to get:
f(0) = 0^2 / 0
Since we get an expression of the form 0/0, we know that we have an indeterminate form. We can try factoring the expression to determine the limit.
Factoring the expression gives us:
f(0) = x^2 / x
= x(x/x)
= x(1)
= 0(1)
= 0
We have successfully determined the limit, which is 0.
Example 6: Determine the limit as x approaches 0 of the function f(x) = sin(x) / x^2.
Using direct substitution, we plug in x = 0 to get:
f(0) = sin(0) / 0^2
= sin(0) / 0
Since we get an expression of the form b/0, where b is not equal to 0, we know that we have an asymptote. We can conclude that the limit does not exist.
Example 7: Determine the limit as x approaches 0 of the function f(x) = (x^2 + 2x + 1) / x.
Using direct substitution, we plug in x = 0 to get:
f(0) = (0^2 + 2*0 + 1) / 0
= (0 + 0 + 1) / 0
Since we get an expression of the form b/0, where b is not equal to 0, we know that we have an asymptote. We can conclude that the limit does not exist.
As you can see, selecting the appropriate method for determining a limit is an important skill in calculus. By starting with the simplest method (direct substitution) and then trying more complex methods if necessary, you can successfully determine the limits of a wide variety of functions. With practice and persistence, you will become proficient at this skill and will be well on your way to success in your studies of calculus.
Browse Study Guides By Unit
👑Unit 1 – Limits & Continuity
🤓Unit 2 – Fundamentals of Differentiation
🤙🏽Unit 3 – Composite, Implicit, & Inverse Functions
👀Unit 4 – Contextual Applications of Differentiation
✨Unit 5 – Analytical Applications of Differentiation
🔥Unit 6 – Integration & Accumulation of Change
💎Unit 7 – Differential Equations
🐶Unit 8 – Applications of Integration
🦖Unit 9 – Parametric Equations, Polar Coordinates, & Vector-Valued Functions (BC Only)
♾Unit 10 – Infinite Sequences & Series (BC Only)
🧐Multiple Choice Questions (MCQ)
✍️Free Response Questions (FRQ)
📆Big Reviews: Finals & Exam Prep

© 2023 Fiveable Inc. All rights reserved.