1.3 Fluids: Pressure and Forces

5 min read•june 18, 2024

Daniella Garcia-Loos

Krish Gupta

Daniella Garcia-Loos

Krish Gupta

AP Physics 2 🧲

61 resources

See Units

Pressure

What is Pressure?

You are probably familiar with the quantity of force, the interaction that causes a change in an object’s motion. In fluid dynamics, we also look at the property of pressure, which is the ratio of the force to the perpendicular area of the object. This is typically measured in atmospheres (atm) 😺

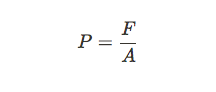
The pressure exerted on an object submerged in a liquid is known as hydrostatic pressure. If we take the weight of the object and divide it by its area, we get the hydrostatic pressure 🚿 which equals pgh. It is important to take notice that the pressure felt by an object only depends on the density of the liquid and how deep the object is submerged from the top of the liquid. It does not depend at all on the mass of the object.

The total, or absolute, pressure an object experiences has 2 components. Gauge pressure, which is due to the liquid, and atmospheric pressure, which is due to the atmosphere 🤓

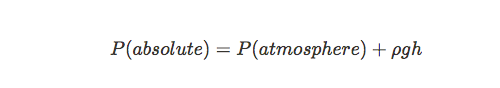
Therefore total pressure equals gauge pressure (pgh) added to the atmospheric pressure. Pay attention to the fact that gauge pressure depends on the density of the fluid and how deep the object is from the top of the fluid. If the atmospheric pressure is not given to you in the problem, you can assume it to be 1 atmosphere.

Here are some key points about the pressure equation:

The pressure equation is a mathematical equation that describes the relationship between the pressure of a fluid and its density, velocity, and height above a reference point.
The general form of the pressure equation is:

P = ρgh + 1/2 * ρv^2

Where P is the pressure, ρ is the density of the fluid, g is the acceleration due to gravity, h is the height above the reference point, and v is the velocity of the fluid.

The first term on the right side of the equation (ρgh) is the hydrostatic pressure, which is the pressure exerted by a static fluid due to its weight.
The second term on the right side of the equation (1/2 * ρv^2) is the dynamic pressure, which is the pressure exerted by a moving fluid due to its kinetic energy.
The pressure equation is used to analyze a variety of fluid flow problems, including fluid flow through pipes and fluid flow over surfaces.
The pressure equation can be used to calculate the pressure at any point in a fluid, given the density, velocity, and height of the fluid at that point.

Q. What is the gauge pressure in an open fish tank if the absolute pressure is 5 atms?

A. Absolute pressure is the sum of the atmosphere and gauge pressure. Since the atmospheric pressure due to air can be assumed to be 1 atmosphere and the absolute pressure is 5 atms the gauge pressure is 5-1= 4 atms.

Caution: atmospheric pressure won't always be 1, especially if you're in a closed or sealed container (like in closed pipes). Atmospheric pressure can be assumed to be one in a container that is open to the environment!

It is also important to keep in mind that unlike force, pressure is a scalar. Pressure is defined to be at the point perpendicular to the surface. Therefore, going left or right across a liquid won’t affect the pressure the object feels.

Pascal's Principle

Pascal’s principle states that pressure at a point in a fluid is equal in all directions. This fact is used to lift heavy objects using hydraulic lifts 🏋️‍♀️ Since the pressures have to be equal, we can apply a small force to a small area and obtain a much larger push back from the liquid over a much larger area.

Here are some key points about Pascal's principle:

Pascal's principle states that the pressure applied to a confined fluid is transmitted undiminished to every part of the fluid and to the walls of the container.
This means that if you apply a force to one part of a confined fluid, the pressure will increase throughout the entire fluid, and the force will be transmitted to every point in the fluid and to the walls of the container.
Pascal's principle is a fundamental principle of fluid mechanics, and it is the basis for many practical devices, including hydraulic lifts, hydraulic brakes, and hydraulic presses.
Pascal's principle can be derived from the laws of physics, specifically the law of conservation of energy and the equation of state for a fluid.
Pascal's principle is often used in conjunction with other principles, such as the principle of buoyancy and the principle of continuity, to analyze and solve problems involving fluid flow.

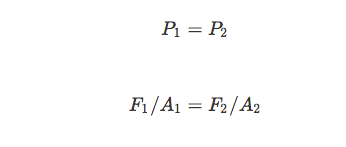
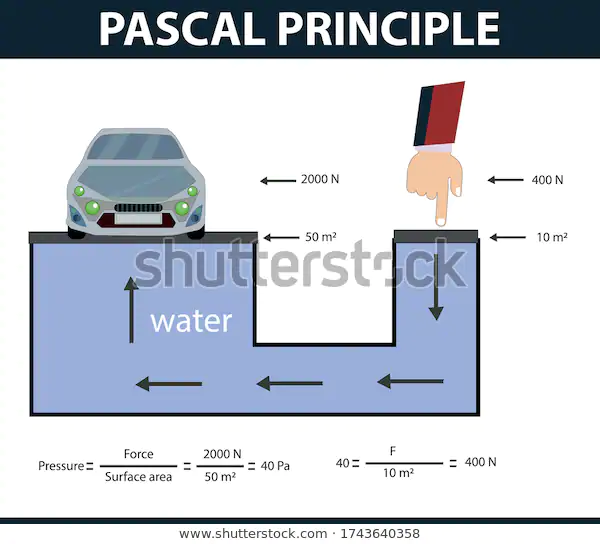
Pascal Principle shown in the image

Pressure and Velocity: The Bernoulli Effect

Pressure and velocity are inversely correlated. This is one of the most asked and frequently missed principles on exams. Areas of low pressure have high velocity, and areas of high pressure have low velocity. Our intuition would tell us that fast-moving things would have more pressure, but, instead, this principle focuses on the pressure on the walls of the container. Slow-moving liquids exert greater pressure on the walls of the container than faster-moving liquids. This is called the Bernoulli effect.

The Bernoulli effect is what leads to air flow and the phenomenon known as lift, allowing for inventions like the airplane. So the next time you fly on an airplane, remember to thank Bernoulli. ✈️

The concept of pressure is first discussed in this unit with regards to fluids, but it also comes up later in the Unit 2 and Thermodynamics. In this section, we mostly focus on the interactions of an object in liquids. Later we will shift our focus to gases.

Here are some key points about the Bernoulli effect:

The Bernoulli effect is a principle of fluid dynamics that states that an increase in the velocity of a fluid results in a decrease in the pressure of the fluid.
The Bernoulli effect is a result of the conservation of energy in a fluid system. As the velocity of the fluid increases, the kinetic energy of the fluid increases, and the potential energy of the fluid decreases. This results in a decrease in the pressure of the fluid.
The Bernoulli effect can be observed in a variety of situations, including fluid flow through pipes, fluid flow over surfaces, and fluid flow through a venturi tube.
The Bernoulli effect is often used to explain the lift generated by an airplane wing and the lift generated by a golf ball.
The Bernoulli effect can be described mathematically using the Bernoulli equation, which relates the pressure, velocity, and height of a fluid in a system.

Browse Study Guides By Unit

💧Unit 1 – Fluids

1.1Fluid Systems

1.2Density

1.3Fluids: Pressure and Forces